Difference between revisions of "User:Jhurley/sandbox"

From Enviro Wiki
Jump to: navigation, search
(→‎Advection-Dispersion-Reaction Equation for Solute Transport)
Line 1: Line 1:
  
−
==Advection-Dispersion-Reaction Equation for Solute Transport==
+
==Matrix Diffusion==
  
−
The transport of dissolved solutes in groundwater is often modeled using the Advection-Dispersion-Reaction (ADR) equation. [[wikipedia:Advection|Advection]] refers to the bulk movement of solutes carried by flowing groundwater. [[wikipedia:Dispersion|Dispersion]] refers to the spreading of the contaminant plume from highly concentrated areas to less concentrated areas. Dispersion coefficients are calculated as the sum of [[wikipedia:Molecular diffusion | molecular diffusion]], mechanical dispersion, and macrodispersion. Reaction refers to changes in the mass of the solute within the system resulting from biotic and abiotic processes.
+
Matrix Diffusion describes the gradual transport of dissolved contaminants from higher concentration and higher hydraulic conductivity (''K'') zones of a heterogeneous aquifer into lower ''K'' and lower contaminant concentration zones by [[wikipedia:Molecular diffusion | molecular diffusion]]. Initially, the transfer of contaminant mass into the low ''K'' zones reduces the concentration in the high ''K'' zones and slows the migration of the plume. Once the contaminant source is removed and the high ''K'' zone contaminant concentration decreases, the contaminants will then diffuse back out of these low ''K'' zones. In some cases, matrix diffusion can maintain contaminant concentrations in more permeable zones at greater than target cleanup goals for decades or even centuries after the primary sources have been addressed<ref name="Chapman2005">Chapman, S.W. and Parker, B.L., 2005. Plume persistence due to aquitard back diffusion following dense nonaqueous phase liquid source removal or isolation. Water Resources Research, 41(12), Report W12411.  [https://doi.org/10.1029/2005WR004224 DOI: 10.1029/2005WR004224] [[Media:Chapman2005.pdf | Report.pdf]] Free access article from [https://agupubs.onlinelibrary.wiley.com/doi/full/10.1029/2005WR004224 American Geophysical Union]</ref>. Field and laboratory results have illustrated the importance of this process.  Analytical and numerical modeling tools are available for evaluating matrix diffusion.
 
<div style="float:right;margin:0 0 2em 2em;">__TOC__</div>
 
<div style="float:right;margin:0 0 2em 2em;">__TOC__</div>
  
−
'''Related Article(s):'''
+
'''Related Article(s): '''
−
*[[Advection and Groundwater Flow]]
+
*[[Advection and Groundwater Flow]]  
 
*[[Dispersion and Diffusion]]
 
*[[Dispersion and Diffusion]]
−
*[[Sorption of Organic Contaminants]]
 
−
*[[Plume Response Modeling]]
 
 
*[[REMChlor - MD]]
 
*[[REMChlor - MD]]
  
−
'''CONTRIBUTOR(S):'''  
+
'''Contributors: '''
 
*[[Dr. Charles Newell, P.E.]]
 
*[[Dr. Charles Newell, P.E.]]
 
*[[Dr. Robert Borden, P.E.]]
 
*[[Dr. Robert Borden, P.E.]]
  
−
'''Key Resource(s):'''
+
'''Key Resource(s): '''
−
*[http://hydrogeologistswithoutborders.org/wordpress/1979-english/ Groundwater]<ref name="FandC1979">Freeze, A., and Cherry, J., 1979. Groundwater, Prentice-Hall, Englewood Cliffs, New Jersey, 604 pages. Free download from [http://hydrogeologistswithoutborders.org/wordpress/1979-english/ Hydrogeologists Without Borders].</ref>, Freeze and Cherry, 1979.
+
*[https://www.serdp-estcp.org/content/download/23838/240653/file/ER-1740 Management of Contaminants Stored in Low Permeability Zones – A State of the Science Review]<ref name="Sale2013">Sale, T., Parker, B.L., Newell, C.J. and Devlin, J.F., 2013. Management of Contaminants Stored in Low Permeability Zones – A State of the Science Review. Strategic Environmental Research and Development Program (SERDP) Project ER-1740. [[Media:Sale2013ER-1740.pdf | Report.pdf]]  Website: [https://www.serdp-estcp.org/Program-Areas/Environmental-Restoration/Contaminated-Groundwater/Persistent-Contamination/ER-1740 ER-1740]</ref>
−
*[https://gw-project.org/books/hydrogeologic-properties-of-earth-materials-and-principles-of-groundwater-flow/ Hydrogeologic Properties of Earth Materials and Principals of Groundwater Flow]<ref name="Woessner2020">Woessner, W.W., and Poeter, E.P., 2020. Properties of Earth Materials and Principals of Groundwater Flow, The Groundwater Project, Guelph, Ontario, 207 pages. Free download from [https://gw-project.org/books/hydrogeologic-properties-of-earth-materials-and-principles-of-groundwater-flow/ The Groundwater Project].</ref>, Woessner and Poeter, 2020.
 
  
−
==The ADR Equation==
+
==Introduction==  
−
[[File:AdvectionFig5.png | thumb | right | 350px | Figure 1. Curves of concentration versus distance (a) and concentration versus time (b) generated by solving the ADR equation for a continuous source of a non-reactive tracer with ''R'' = 1, λ = 0, ''v'' = 5 m/yr, and ''D<sub>x</sub>'' = 10 m<sup>2</sup>/yr.]]
+
[[File:NewellMatrixDiffFig1.PNG | thumb |500px| Figure 1. Diffusion of a dissolved solute (chlorinated solvent) into lower ''K'' zones during loading period, followed by diffusion back out into higher ''K'' zones once the source is removed <ref name="Sale2007">Sale, T.C., Illangasekare, T.H., Zimbron, J., Rodriguez, D., Wilking, B., and Marinelli, F., 2007. AFCEE Source Zone Initiative. Air Force Center for Environmental Excellence, Brooks City-Base, San Antonio, TX. [https://www.enviro.wiki/images/0/08/AFCEE-2007-Sale.pdf Report.pdf]</ref>]]
−
[[File:ADRFig2.PNG | thumb | right | 350px | Figure 2. Predicted variation in macrodispersivity with distance for varying ''σ<sup>2</sup>Y'' and correlation length = 3 m.]]
+
Matrix Diffusion can have major impacts on solute migration in groundwater and on cleanup time following source removal.  As a groundwater plume advances downgradient, dissolved contaminants are transported by molecular diffusion from zones with larger hydraulic conductivity (''K'') into lower ''K'' zones, slowing the rate of contaminant migration in the high ''K'' zone. However, once the contaminant source is eliminated, contaminants diffuse out of low ''K'' zones, slowing the cleanup rate in the high ''K'' zone (Figure 1).  This process, termed ‘back diffusion’, can greatly extend cleanup times.
−
In many groundwater transport models, solute transport is described by the advection-dispersion-reaction equation. As shown below (Equation 1), the ADR equation describes the change in dissolved solute concentration (''C'') over time (''t'') where groundwater flow is oriented along the ''x'' direction.
 
  
−
{|
+
==Lab-Scale Studies==
−
| || [[File:AdvectionEq3r.PNG|center|635px]]
+
The impacts of back diffusion on aquifer cleanup have been examined in controlled laboratory experiments by several investigators<ref name="Doner2008">Doner, L.A., 2008. Tools to resolve water quality benefits of upgradient contaminant flux reduction. Master’s Thesis, Department of Civil and Environmental Engineering, Colorado State University.</ref><ref name="Yang2015">Yang, M., Annable, M.D. and Jawitz, J.W., 2015. Back Diffusion from Thin Low Permeability Zones. Environmental Science and Technology, 49(1), pp. 415-422.  [https://doi.org/10.1021/es5045634 DOI: 10.1021/es5045634] Free download available from: [https://www.researchgate.net/publication/269189924_Back_Diffusion_from_Thin_Low_Permeability_Zones ResearchGate]</ref><ref name= "Yang2016">Yang, M., Annable, M.D. and Jawitz, J.W., 2016. Solute source depletion control of forward and back diffusion through low-permeability zones. Journal of Contaminant Hydrology, 193, pp. 54-62. [https://doi.org/10.1016/j.jconhyd.2016.09.004 DOI: 10.1016/j.jconhyd.2016.09.004] Free download available from: [https://www.researchgate.net/profile/Minjune_Yang/publication/308004091_Solute_source_depletion_control_of_forward_and_back_diffusion_through_low-permeability_zones/links/5a2ed2c44585155b6179f489/Solute-source-depletion-control-of-forward-and-back-diffusion-through-low-permeability-zones.pdf ResearchGate]</ref><ref name="Tatti2018">Tatti, F., Papini, M.P., Sappa, G., Raboni, M., Arjmand, F., and Viotti, P., 2018. Contaminant back-diffusion from low-permeability layers as affected by groundwater velocity: A laboratory investigation by box model and image analysis. Science of The Total Environment, 622, pp. 164-171. [https://doi.org/10.1016/j.scitotenv.2017.11.347 DOI: 10.1016/j.scitotenv.2017.11.347]</ref>.  The video in Figure 2 shows the results of a 122-day tracer test in a laboratory flow cell (sand box)<ref name="Doner2008"/>.  The flow cell contained several clay zones (''K'' = 10<sup>-8</sup> cm/s) surrounded by sand (''K'' = 0.02 cm/s).  During the loading period, water containing a green fluorescent tracer migrates from left to right with the water flowing through the flow cell, while diffusing into the clay.  After 22 days, the fluorescent tracer is eliminated from the feed, and most of the green tracer is quickly flushed from the tank’s sandy zones.  However, small amounts of tracer continue to diffuse out of the clay layers for over 100 days.  This illustrates how back diffusion of contaminants out of low ''K'' zones can maintain low contaminant concentrations long after the contaminant source as been eliminated.
 +
 
 +
[[File: GreenTank.mp4 |thumbnail|500px| Figure 2. Video of dye tank simulation of matrix diffusion]]
 +
==Field Studies==
 +
In some cases, matrix diffusion can maintain contaminant concentrations in more permeable zones above target cleanup goals for decades or even centuries after the primary sources have been addressed.  At a site impacted by Dense Non-Aqueous Phase Liquids (DNAPL), [[Chlorinated Solvents | trichloroethene (TCE)]] concentrations in downgradient wells declined by roughly an order-of-magnitude (OoM), when the upgradient source area was isolated with sheet piling.  However, after this initial decline, TCE concentrations appeared to plateau or decline more slowly, consistent with back diffusion from an underlying aquitard.  Numerical simulations indicated that back diffusion would cause TCE concentrations in downgradient wells at the site to remain above target cleanup levels for centuries<ref name="Chapman2005"/>.
 +
 
 +
One other implication of matrix diffusion is that plume migration is attenuated by the loss of contaminants into low permeability zones, leading to slower plume migration compared to a case where no matrix diffusion occurs.  This phenomena was observed as far back as 1985 when Sudicky et al. observed that “A second consequence of the solute-storage effect offered by transverse diffusion into low-permeability layers is a rate of migration of the frontal portion of a contaminant in the permeable layers that is less than the groundwater velocity.”<ref name="Sudicky1985"> Sudicky, E.A., Gillham, R.W., and Frind, E.O., 1985. Experimental Investigation of Solute Transport in Stratified Porous Media: 1. The Nonreactive Case. Water Resources Research, 21(7), pp. 1035-1041. [https://doi.org/10.1029/WR021i007p01035 DOI: 10.1029/WR021i007p01035]</ref>  In cases where there is an attenuating source, matrix diffusion can also reduce the peak concentrations observed in downgradient monitoring wells.  The attenuation caused by matrix diffusion may be particularly important for implementing [[Monitored Natural Attenuation (MNA)]] for contaminants that do not completely degrade, such as [[Metal and Metalloid Contaminants | heavy metals]] and [[Perfluoroalkyl_and_Polyfluoroalkyl_Substances_(PFAS) | PFAS]].
 +
 
 +
==SERPD/ESTCP Research==
 +
{|
 +
The SERDP/ESTCP programs have funded several projects focusing on how matrix diffusion can impede progress towards reaching site closure, including:
 
|-
 
|-
−
| Where: ||
+
|
 +
*[https://www.serdp-estcp.org/Program-Areas/Environmental-Restoration/Contaminated-Groundwater/Persistent-Contamination/ER-1740 SERDP Management of Contaminants Stored in Low Permeability Zones, A State-of-the-Science Review] <ref name="Sale2013"/>
 
|-
 
|-
 
|  
 
|  
−
:''R''
+
*[https://www.serdp-estcp.org/Tools-and-Training/Environmental-Restoration/Groundwater-Plume-Treatment/Matrix-Diffusion-Tool-Kit ESTCP Matrix Diffusion Toolkit]<ref name="Farhat2012">Farhat, S.K., Newell, C.J., Seyedabbasi, M.A., McDade, J.M., Mahler, N.T., Sale, T.C., Dandy, D.S. and Wahlberg, J.J., 2012. Matrix Diffusion Toolkit. Environmental Security Technology Certification Program (ESTCP) Project ER-201126.  [[Media:Farhat2012ER-201126UsersManual.pdf | User’s Manual.pdf]]  Website: [https://www.serdp-estcp.org/Tools-and-Training/Environmental-Restoration/Groundwater-Plume-Treatment/Matrix-Diffusion-Tool-Kit ER-201126]</ref>
−
| is the linear, equilibrium retardation factor (see [[Sorption of Organic Contaminants]]),  
 
 
|-
 
|-
 
|  
 
|  
−
:''D<sub>x</sub>, D<sub>y</sub>, and D<sub>z</sub>''&nbsp;&nbsp;
+
*[https://www.serdp-estcp.org/Program-Areas/Environmental-Restoration/Contaminated-Groundwater/Persistent-Contamination/ER-200530 ESTCP Decision Guide]<ref>Sale, T. and Newell, C., 2011. A Guide for Selecting Remedies for Subsurface Releases of Chlorinated Solvents. Environmental Security Technology Certification Program (ESTCP) Project ER-200530. [[Media: Sale2011ER-200530.pdf | Report.pdf]]  Website: [https://www.serdp-estcp.org/Program-Areas/Environmental-Restoration/Contaminated-Groundwater/Persistent-Contamination/ER-200530 ER-200530]</ref>
−
| are hydrodynamic dispersion coefficients in the ''x, y'' and ''z'' directions (L<sup>2</sup>/T),
 
 
|-
 
|-
−
|  
+
|
−
:''v''
+
*[https://www.serdp-estcp.org/Program-Areas/Environmental-Restoration/Contaminated-Groundwater/Persistent-Contamination/ER-201426 ESTCP REMChlor-MD: the USEPA’s REMChlor model with a new matrix diffusion term for the plume]<ref name="Farhat2018">Farhat, S. K., Newell, C. J., Falta, R. W., and Lynch, K., 2018. A Practical Approach for Modeling Matrix Diffusion Effects in REMChlor. Environmental Security Technology Certification Program (ESTCP) Project ER-201426.  [https://enviro.wiki/images/0/0b/2018-Falta-REMChlor_Modeling_Matrix_Diffusion_Effects.pdf  User’s Manual.pdf]  Website: [https://www.serdp-estcp.org/Program-Areas/Environmental-Restoration/Contaminated-Groundwater/Persistent-Contamination/ER-201426 ER-201426]</ref>
−
| is the advective transport or seepage velocity in the ''x'' direction (L/T), and
 
−
|-
 
−
|
 
−
:''λ''
 
−
| is an effective first order decay rate due to combined biotic and abiotic processes (1/T).
 
 
|}
 
|}
−
The term on the left side of the equation is the rate of mass change per unit volume.  On the right side are terms representing the solute flux due to dispersion in the ''x, y'', and ''z'' directions, the advective flux in the ''x'' direction, and the first order decay due to biotic and abiotic processes. Dispersion coefficients (''D<sub>x,y,z</sub>'') are commonly estimated using the following relationships:
 
−
 
−
{|
 
−
| || [[File:AdvectionEq4.PNG|center|360px]]
 
−
|-
 
−
| Where: ||
 
−
|-
 
−
|
 
−
:''D<sub>m</sub>''
 
−
| is the molecular diffusion coefficient (L<sup>2</sup>/T), and
 
−
|-
 
−
|
 
−
:''&alpha;<sub>L</sub>, &alpha;<sub>T</sub>'', and ''&alpha;<sub>V</sub>''&nbsp;&nbsp;
 
−
| are the longitudinal, transverse and vertical dispersivities (L).
 
−
|}
 
−
Figures 1a and 1b were generated using a numerical solution of the ADR equation for a non-reactive tracer (''R'' = 1; λ = 0) with ''v'' = 5 m/yr and ''D<sub>x</sub>'' = 10 m<sup>2</sup>/yr. 
 
−
Figure 1a shows the predicted change in concentration of the tracer, chloride, versus distance downgradient from the continuous contaminant source at different times (0, 1, 2, and 4 years).  Figure 1b shows the change in concentration versus time (commonly referred to as the breakthrough curve or BTC) at different downgradient distances (10, 20, 30 and 40 m).  At 2 years, the mid-point of the concentration versus distance curve (Figure 1a) is located 10 m downgradient (x = 5 m/yr * 2 yr).  At 20 m downgradient, the mid-point of the concentration versus time curves (Figure 1b) occurs at 4 years (t = 20 m / 5 m/yr).
 
−
 
−
==Modeling Dispersion==
 
−
The dispersion coefficient in the ADR equation accounts for the combined effects of molecular diffusion and mechanical dispersion, both of which cause spreading of the contaminant plume from highly concentrated areas toward less concentrated areas.  [[wikipedia:Molecular diffusion | Molecular diffusion]] is the result of the thermal motion of individual molecules which causes a flux of dissolved solutes from areas of higher concentration to areas of lower concentration.  Mechanical dispersion (hydrodynamic dispersion) results from groundwater moving at rates that vary from the average linear velocity. Because the invading solute-containing water does not travel at the same velocity everywhere, mixing occurs along flow paths. Typical values of the mechanical dispersivity measured in laboratory column tests are on the order of 0.01 to 1 cm<ref name="Anderson1979">Anderson, M.P. and Cherry, J.A., 1979. Using models to simulate the movement of contaminants through groundwater flow systems. Critical Reviews in Environmental Science and Technology, 9(2), pp.97-156.  [https://doi.org/10.1080/10643387909381669 DOI: 10.1080/10643387909381669]</ref>.
 
−
 
−
Spatial variations in hydraulic conductivity can increase the apparent spreading of solute plumes observed in groundwater monitoring wells. This spreading of the solute caused by large-scale heterogeneities in the aquifer and the associated spatial variations in advective transport velocity is referred to as macrodispersion. In some groundwater modeling projects, large values of dispersivity are used as an adjustment factor to better represent the observed large-scale spreading of plumes<ref name="ITRC2015">Interstate Technology and Regulatory Council (ITRC), 2015. Integrated DNAPL Site Characterization and Tools Selection (ISC-1), DNAPL Site Characterization Team, ITRC, Washington, DC. [[Media:ITRC2015-ISC-1.pdf | Report.pdf]] Free download from: [https://www.itrcweb.org/DNAPL-ISC_tools-selection/Content/Resources/DNAPLPDF.pdf ITRC]</ref>. Theoretical studies suggest that macrodispersivity will increase with distance near the source, and then increase more slowly farther downgradient, eventually approaching an asymptotic value<ref name="Gelhar1979">Gelhar, L.W., Gutjahr, A.L. and Naff, R.L., 1979. Stochastic analysis of macrodispersion in a stratified aquifer. Water Resources Research, 15(6), pp.1387-1397.  [https://doi.org/10.1029/WR015i006p01387 DOI:10.1029/WR015i006p01387]</ref><ref name="Gelhar1983">Gelhar, L.W. and Axness, C.L., 1983. Three‐dimensional stochastic analysis of macrodispersion in aquifers. Water Resources Research, 19(1), pp.161-180.  [https://doi.org/10.1029/WR019i001p00161 DOI:10.1029/WR019i001p00161]</ref><ref name="Dagan1988">Dagan, G., 1988. Time‐dependent macrodispersion for solute transport in anisotropic heterogeneous aquifers. Water Resources Research, 24(9), pp.1491-1500.  [https://doi.org/10.1029/WR024i009p01491 DOI:10.1029/WR024i009p01491]</ref>.  Figure 2 shows values of macrodispersivity calculated using the theory of Dagan<ref name="Dagan1988"/> with an autocorrelation length of 3 m and several different values of the variance of ''Y'' (σ<small><sup>2</sup><sub>''Y''</sub></small>) where ''Y'' = Log ''K''. The calculated macrodispersivity increases more rapidly and reaches higher asymptotic values for more heterogeneous aquifers with greater variations in ''K'' (larger σ<small><sup>2</sup><sub>''Y''</sub></small>).  The maximum predicted dispersivity values are in the range of 0.5 to 5 m.
 
−
 
−
==Modeling Diffusion==
 
−
[[File:ADRFig3.png | thumb| 350px| Figure 3.  Comparison of tracer breakthrough (upper graph) and cleanup curves (lower graph) from advection-dispersion based (gray lines) and advection-diffusion based (black lines) solute transport<ref name="ITRC2011">Interstate Technology and Regulatory Council (ITRC), 2011. Integrated DNAPL Site Strategy (IDSS-1),  Integrated DNAPL Site Strategy Team, ITRC, Washington, DC. [https://www.enviro.wiki/images/d/d9/ITRC-2011-Integrated_DNAPL.pdf Report.pdf]  Free download from:
 
−
[https://itrcweb.org/GuidanceDocuments/IntegratedDNAPLStrategy_IDSSDoc/IDSS-1.pdf ITRC]</ref>.]]
 
−
[[Matrix Diffusion]] describes the gradual transport  of dissolved contaminants from higher concentration and higher hydraulic conductivity (''K'') zones into low ''K'' zones by [[wikipedia:Molecular diffusion | molecular diffusion]]. The contaminants can then diffuse back out of these low ''K'' zones once the contaminant source is removed and the high ''K'' zone contaminant concentration decreases.  In some cases, matrix diffusion can maintain contaminant concentrations in more permeable zones at greater than target cleanup goals for decades or even centuries after the primary sources have been addressed<ref name="Chapman2005">Chapman, S.W. and Parker, B.L., 2005. Plume persistence due to aquitard back diffusion following dense nonaqueous phase liquid source removal or isolation. Water Resources Research, 41(12), Report W12411.  [https://doi.org/10.1029/2005WR004224 DOI: 10.1029/2005WR004224] [[Media:Chapman2005.pdf | Report.pdf]]  Free access article from [https://agupubs.onlinelibrary.wiley.com/doi/full/10.1029/2005WR004224 American Geophysical Union]</ref>. (See also [[Matrix Diffusion]].)
 
−
 
−
===Impacts on Breakthrough Curves===
 
−
The impacts of matrix diffusion on the initial breakthrough of the solute plume and on later cleanup are illustrated in Figure 3<ref name="ITRC2011"/>. Using a traditional advection-dispersion model, the breakthrough curve for a pulse tracer injection appears as a bell-shaped ([[wikipedia:Gaussian function |Gaussian]]) curve (gray line on the right side of the upper graph) where the peak arrival time corresponds to the average groundwater velocity.  Using an advection-diffusion approach, the breakthrough curve for a pulse injection is asymmetric (solid black line) with the peak tracer concentration arriving earlier than would be expected based on the average groundwater velocity, but with a long extended tail to the flushout curve.
 
  
−
The lower graph shows the predicted cleanup concentration profiles following complete elimination of a source area.  The advection-dispersion model (gray line) predicts a clean-water front arriving at a time corresponding to the average groundwater velocity. The advection-diffusion model (black line) predicts that concentrations will start to decline more rapidly than expected (based on the average groundwater velocity) as clean water rapidly migrates through the highest-permeability strata. However, low but significant contaminant concentrations linger much longer (tailing) due to diffusive contaminant mass exchange between zones of high and low permeability.
+
==Modeling Matrix Diffusion==
 +
Several different modeling approaches have been developed to simulate the diffusive transport of dissolved solutes into and out of lower ''K'' zones.  The Matrix Diffusion Toolkit<ref name="Farhat2012"/> is a Microsoft Excel based tool for simulating forward and back diffusion using two different analytical models<ref name="Parker1994">Parker, B.L., Gillham, R.W., and Cherry, J.A., 1994. Diffusive Disappearance of Immiscible Phase Organic Liquids in Fractured Geologic Media. Groundwater, 32(5), pp. 805-820. [https://doi.org/10.1111/j.1745-6584.1994.tb00922.x DOI: 10.1111/j.1745-6584.1994.tb00922.x]</ref><ref>Sale, T.C., Zimbron, J.A., and Dandy, D.S., 2008. Effects of reduced contaminant loading on downgradient water quality in an idealized two-layer granular porous media. Journal of Contaminant Hydrology, 102(1), pp. 72-85. [https://doi.org/10.1016/j.jconhyd.2008.08.002 DOI: 10.1016/j.jconhyd.2008.08.002]</ref>.  Numerical models including [https://www.usgs.gov/software/mt3d-usgs-groundwater-solute-transport-simulator-modflow MODFLOW/MT3DMS]<ref name="Zheng1999">Zheng, C. and Wang, P.P., 1999. MT3DMS: A Modular Three-Dimensional Multispecies Transport Model for Simulation of Advection, Dispersion, and Chemical Reactions of Contaminants in Groundwater Systems; Documentation and User’s Guide. Contract Report SERDP-99-1 U.S. Army Engineer Research and Development Center, Vicksburg, MS. [https://www.enviro.wiki/images/3/32/Mt3dmanual.pdf User’s Guide.pdf]  [https://www.usgs.gov/software/mt3d-usgs-groundwater-solute-transport-simulator-modflow MT3DMS website]</ref> have been shown to be effective in simulating back diffusion process and can accurately predict concentration changes over 3 orders-of-magnitude in heterogeneous sand tank experiments<ref>Chapman, S.W., Parker, B.L., Sale, T.C., Doner, L.A., 2012. Testing high resolution numerical models for analysis of contaminant storage and release from low permeability zones. Journal of Contaminant Hydrology, 136, pp. 106-116. [https://doi.org/10.1016/j.jconhyd.2012.04.006 DOI: 10.1016/j.jconhyd.2012.04.006]</ref>. However, numerical models require a fine vertical discretization with short time steps to accurately simulate back diffusion, greatly increasing computation times<ref>Farhat, S.K., Adamson, D.T., Gavaskar, A.R., Lee, S.A., Falta, R.W. and Newell, C.J., 2020. Vertical Discretization Impact in Numerical Modeling of Matrix Diffusion in Contaminated Groundwater. Groundwater Monitoring and Remediation, 40(2), pp. 52-64. [https://doi.org/10.1111/gwmr.12373 DOI: 10.1111/gwmr.12373]</ref>.  These issues can be addressed by incorporating a local 1-D model domain within a general 3D numerical model<ref>Carey, G.R., Chapman, S.W., Parker, B.L. and McGregor, R., 2015. Application of an Adapted Version of MT3DMS for Modeling Back‐Diffusion Remediation Timeframes. Remediation, 25(4), pp. 55-79. [https://doi.org/10.1002/rem.21440 DOI: 10.1002/rem.21440]</ref>.
  
−
==ADR Applications==
+
The [[REMChlor - MD]] toolkit is capable of simulating matrix diffusion in groundwater contaminant plumes by using a semi-analytical method for estimating mass transfer between high and low permeability zones that provides computationally accurate predictions, with much shorter run times than traditional fine grid numerical models<ref name="Farhat2018"/>.
−
The ADR equation can be solved to find the spatial and temporal distribution of solutes using a variety of analytical and numerical approaches.  The design tools [https://www.epa.gov/water-research/bioscreen-natural-attenuation-decision-support-system BIOSCREEN]<ref name="Newell1996">Newell, C.J., McLeod, R.K. and Gonzales, J.R., 1996. BIOSCREEN: Natural Attenuation Decision Support System - User's Manual, Version 1.3. US Environmental Protection Agency, EPA/600/R-96/087. [https://www.enviro.wiki/index.php?title=File:Newell-1996-Bioscreen_Natural_Attenuation_Decision_Support_System.pdf Report.pdf]  [https://www.epa.gov/water-research/bioscreen-natural-attenuation-decision-support-system BIOSCREEN website]</ref>, [https://www.epa.gov/water-research/biochlor-natural-attenuation-decision-support-system BIOCHLOR]<ref name="Aziz2000">Aziz, C.E., Newell, C.J., Gonzales, J.R., Haas, P.E., Clement, T.P. and Sun, Y., 2000. BIOCHLOR Natural Attenuation Decision Support System. User’s Manual - Version 1.0. US Environmental Protection Agency, EPA/600/R-00/008.  [https://www.enviro.wiki/index.php?title=File:Aziz-2000-BIOCHLOR-Natural_Attenuation_Dec_Support.pdf Report.pdf]  [https://www.epa.gov/water-research/biochlor-natural-attenuation-decision-support-system BIOCHLOR website]</ref>, and [https://www.epa.gov/water-research/remediation-evaluation-model-chlorinated-solvents-remchlor REMChlor]<ref name="Falta2007">Falta, R.W., Stacy, M.B., Ahsanuzzaman, A.N.M., Wang, M. and Earle, R.C., 2007. REMChlor Remediation Evaluation Model for Chlorinated Solvents - User’s Manual, Version 1.0. US Environmental Protection Agency. Center for Subsurface Modeling Support, Ada, OK.  [[Media:REMChlorUserManual.pdf | Report.pdf]]  [https://www.epa.gov/water-research/remediation-evaluation-model-chlorinated-solvents-remchlor REMChlor website]</ref> (see also [[REMChlor - MD]]) employ an analytical solution of the ADR equation.  [https://www.usgs.gov/software/mt3d-usgs-groundwater-solute-transport-simulator-modflow MT3DMS]<ref name="Zheng1999">Zheng, C. and Wang, P.P., 1999. MT3DMS: A Modular Three-Dimensional Multispecies Transport Model for Simulation of Advection, Dispersion, and Chemical Reactions of Contaminants in Groundwater Systems; Documentation and User’s Guide. Contract Report SERDP-99-1 U.S. Army Engineer Research and Development Center, Vicksburg, MS. [[Media:Mt3dmanual.pdf | Report.pdf]]  [https://www.usgs.gov/software/mt3d-usgs-groundwater-solute-transport-simulator-modflow MT3DMS website]</ref> uses a numerical method to solve the ADR equation using the head distribution generated by the groundwater flow model MODFLOW<ref name="McDonald1988">McDonald, M.G. and Harbaugh, A.W., 1988. A Modular Three-dimensional Finite-difference Ground-water Flow Model, Techniques of Water-Resources Investigations, Book 6, Modeling Techniques. U.S. Geological Survey, 586 pages. [https://doi.org/10.3133/twri06A1  DOI: 10.3133/twri06A1]  [[Media: McDonald1988.pdf | Report.pdf]]  Free MODFLOW download from: [https://www.usgs.gov/mission-areas/water-resources/science/modflow-and-related-programs?qt-science_center_objects=0#qt-science_center_objects USGS]</ref>.
 
  
 
==References==
 
==References==

Revision as of 21:54, 18 September 2020

Matrix Diffusion

Matrix Diffusion describes the gradual transport of dissolved contaminants from higher concentration and higher hydraulic conductivity (K) zones of a heterogeneous aquifer into lower K and lower contaminant concentration zones by molecular diffusion. Initially, the transfer of contaminant mass into the low K zones reduces the concentration in the high K zones and slows the migration of the plume. Once the contaminant source is removed and the high K zone contaminant concentration decreases, the contaminants will then diffuse back out of these low K zones. In some cases, matrix diffusion can maintain contaminant concentrations in more permeable zones at greater than target cleanup goals for decades or even centuries after the primary sources have been addressed[1]. Field and laboratory results have illustrated the importance of this process. Analytical and numerical modeling tools are available for evaluating matrix diffusion.

Related Article(s):

Contributors:

Key Resource(s):

Introduction

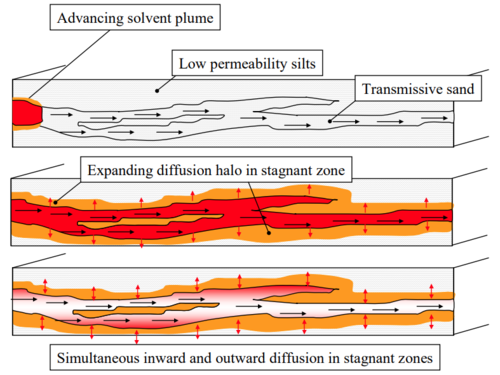
Figure 1. Diffusion of a dissolved solute (chlorinated solvent) into lower K zones during loading period, followed by diffusion back out into higher K zones once the source is removed [3]

Matrix Diffusion can have major impacts on solute migration in groundwater and on cleanup time following source removal. As a groundwater plume advances downgradient, dissolved contaminants are transported by molecular diffusion from zones with larger hydraulic conductivity (K) into lower K zones, slowing the rate of contaminant migration in the high K zone. However, once the contaminant source is eliminated, contaminants diffuse out of low K zones, slowing the cleanup rate in the high K zone (Figure 1). This process, termed ‘back diffusion’, can greatly extend cleanup times.

Lab-Scale Studies

The impacts of back diffusion on aquifer cleanup have been examined in controlled laboratory experiments by several investigators[4][5][6][7]. The video in Figure 2 shows the results of a 122-day tracer test in a laboratory flow cell (sand box)[4]. The flow cell contained several clay zones (K = 10-8 cm/s) surrounded by sand (K = 0.02 cm/s). During the loading period, water containing a green fluorescent tracer migrates from left to right with the water flowing through the flow cell, while diffusing into the clay. After 22 days, the fluorescent tracer is eliminated from the feed, and most of the green tracer is quickly flushed from the tank’s sandy zones. However, small amounts of tracer continue to diffuse out of the clay layers for over 100 days. This illustrates how back diffusion of contaminants out of low K zones can maintain low contaminant concentrations long after the contaminant source as been eliminated.

Figure 2. Video of dye tank simulation of matrix diffusion

Field Studies

In some cases, matrix diffusion can maintain contaminant concentrations in more permeable zones above target cleanup goals for decades or even centuries after the primary sources have been addressed. At a site impacted by Dense Non-Aqueous Phase Liquids (DNAPL), trichloroethene (TCE) concentrations in downgradient wells declined by roughly an order-of-magnitude (OoM), when the upgradient source area was isolated with sheet piling. However, after this initial decline, TCE concentrations appeared to plateau or decline more slowly, consistent with back diffusion from an underlying aquitard. Numerical simulations indicated that back diffusion would cause TCE concentrations in downgradient wells at the site to remain above target cleanup levels for centuries[1].

One other implication of matrix diffusion is that plume migration is attenuated by the loss of contaminants into low permeability zones, leading to slower plume migration compared to a case where no matrix diffusion occurs. This phenomena was observed as far back as 1985 when Sudicky et al. observed that “A second consequence of the solute-storage effect offered by transverse diffusion into low-permeability layers is a rate of migration of the frontal portion of a contaminant in the permeable layers that is less than the groundwater velocity.”[8] In cases where there is an attenuating source, matrix diffusion can also reduce the peak concentrations observed in downgradient monitoring wells. The attenuation caused by matrix diffusion may be particularly important for implementing Monitored Natural Attenuation (MNA) for contaminants that do not completely degrade, such as heavy metals and PFAS.

SERPD/ESTCP Research

The SERDP/ESTCP programs have funded several projects focusing on how matrix diffusion can impede progress towards reaching site closure, including:

Modeling Matrix Diffusion

Several different modeling approaches have been developed to simulate the diffusive transport of dissolved solutes into and out of lower K zones. The Matrix Diffusion Toolkit[9] is a Microsoft Excel based tool for simulating forward and back diffusion using two different analytical models[12][13]. Numerical models including MODFLOW/MT3DMS[14] have been shown to be effective in simulating back diffusion process and can accurately predict concentration changes over 3 orders-of-magnitude in heterogeneous sand tank experiments[15]. However, numerical models require a fine vertical discretization with short time steps to accurately simulate back diffusion, greatly increasing computation times[16]. These issues can be addressed by incorporating a local 1-D model domain within a general 3D numerical model[17].

The REMChlor - MD toolkit is capable of simulating matrix diffusion in groundwater contaminant plumes by using a semi-analytical method for estimating mass transfer between high and low permeability zones that provides computationally accurate predictions, with much shorter run times than traditional fine grid numerical models[11].

References

  1. ^ 1.0 1.1 Chapman, S.W. and Parker, B.L., 2005. Plume persistence due to aquitard back diffusion following dense nonaqueous phase liquid source removal or isolation. Water Resources Research, 41(12), Report W12411. DOI: 10.1029/2005WR004224 Report.pdf Free access article from American Geophysical Union
  2. ^ 2.0 2.1 Sale, T., Parker, B.L., Newell, C.J. and Devlin, J.F., 2013. Management of Contaminants Stored in Low Permeability Zones – A State of the Science Review. Strategic Environmental Research and Development Program (SERDP) Project ER-1740. Report.pdf Website: ER-1740
  3. ^ Sale, T.C., Illangasekare, T.H., Zimbron, J., Rodriguez, D., Wilking, B., and Marinelli, F., 2007. AFCEE Source Zone Initiative. Air Force Center for Environmental Excellence, Brooks City-Base, San Antonio, TX. Report.pdf
  4. ^ 4.0 4.1 Doner, L.A., 2008. Tools to resolve water quality benefits of upgradient contaminant flux reduction. Master’s Thesis, Department of Civil and Environmental Engineering, Colorado State University.
  5. ^ Yang, M., Annable, M.D. and Jawitz, J.W., 2015. Back Diffusion from Thin Low Permeability Zones. Environmental Science and Technology, 49(1), pp. 415-422. DOI: 10.1021/es5045634 Free download available from: ResearchGate
  6. ^ Yang, M., Annable, M.D. and Jawitz, J.W., 2016. Solute source depletion control of forward and back diffusion through low-permeability zones. Journal of Contaminant Hydrology, 193, pp. 54-62. DOI: 10.1016/j.jconhyd.2016.09.004 Free download available from: ResearchGate
  7. ^ Tatti, F., Papini, M.P., Sappa, G., Raboni, M., Arjmand, F., and Viotti, P., 2018. Contaminant back-diffusion from low-permeability layers as affected by groundwater velocity: A laboratory investigation by box model and image analysis. Science of The Total Environment, 622, pp. 164-171. DOI: 10.1016/j.scitotenv.2017.11.347
  8. ^ Sudicky, E.A., Gillham, R.W., and Frind, E.O., 1985. Experimental Investigation of Solute Transport in Stratified Porous Media: 1. The Nonreactive Case. Water Resources Research, 21(7), pp. 1035-1041. DOI: 10.1029/WR021i007p01035
  9. ^ 9.0 9.1 Farhat, S.K., Newell, C.J., Seyedabbasi, M.A., McDade, J.M., Mahler, N.T., Sale, T.C., Dandy, D.S. and Wahlberg, J.J., 2012. Matrix Diffusion Toolkit. Environmental Security Technology Certification Program (ESTCP) Project ER-201126. User’s Manual.pdf Website: ER-201126
  10. ^ Sale, T. and Newell, C., 2011. A Guide for Selecting Remedies for Subsurface Releases of Chlorinated Solvents. Environmental Security Technology Certification Program (ESTCP) Project ER-200530. Report.pdf Website: ER-200530
  11. ^ 11.0 11.1 Farhat, S. K., Newell, C. J., Falta, R. W., and Lynch, K., 2018. A Practical Approach for Modeling Matrix Diffusion Effects in REMChlor. Environmental Security Technology Certification Program (ESTCP) Project ER-201426. User’s Manual.pdf Website: ER-201426
  12. ^ Parker, B.L., Gillham, R.W., and Cherry, J.A., 1994. Diffusive Disappearance of Immiscible Phase Organic Liquids in Fractured Geologic Media. Groundwater, 32(5), pp. 805-820. DOI: 10.1111/j.1745-6584.1994.tb00922.x
  13. ^ Sale, T.C., Zimbron, J.A., and Dandy, D.S., 2008. Effects of reduced contaminant loading on downgradient water quality in an idealized two-layer granular porous media. Journal of Contaminant Hydrology, 102(1), pp. 72-85. DOI: 10.1016/j.jconhyd.2008.08.002
  14. ^ Zheng, C. and Wang, P.P., 1999. MT3DMS: A Modular Three-Dimensional Multispecies Transport Model for Simulation of Advection, Dispersion, and Chemical Reactions of Contaminants in Groundwater Systems; Documentation and User’s Guide. Contract Report SERDP-99-1 U.S. Army Engineer Research and Development Center, Vicksburg, MS. User’s Guide.pdf MT3DMS website
  15. ^ Chapman, S.W., Parker, B.L., Sale, T.C., Doner, L.A., 2012. Testing high resolution numerical models for analysis of contaminant storage and release from low permeability zones. Journal of Contaminant Hydrology, 136, pp. 106-116. DOI: 10.1016/j.jconhyd.2012.04.006
  16. ^ Farhat, S.K., Adamson, D.T., Gavaskar, A.R., Lee, S.A., Falta, R.W. and Newell, C.J., 2020. Vertical Discretization Impact in Numerical Modeling of Matrix Diffusion in Contaminated Groundwater. Groundwater Monitoring and Remediation, 40(2), pp. 52-64. DOI: 10.1111/gwmr.12373
  17. ^ Carey, G.R., Chapman, S.W., Parker, B.L. and McGregor, R., 2015. Application of an Adapted Version of MT3DMS for Modeling Back‐Diffusion Remediation Timeframes. Remediation, 25(4), pp. 55-79. DOI: 10.1002/rem.21440

See Also

Retrieved from "https://www.enviro.wiki/index.php?title=User:Jhurley/sandbox&oldid=13974"